Series AFT CCSS Math: Multiplying Whole Numbers & Fractions
Math.Practice.MP2
| Common core State Standards
- Math: Math
- Practice: Mathematical Practice Standards
-
MP2: Reason abstractly and quantitatively.
Mathematically proficient students make sense of quantities and their relationships in problem situations. They bring two complementary abilities to bear on problems involving quantitative relationships: the ability to decontextualize--to abstract a given situation and represent it symbolically and manipulate the representing symbols as if they have a life of their own, without necessarily attending to their referents—and the ability to contextualize, to pause as needed during the manipulation process in order to probe into the referents for the symbols involved. Quantitative reasoning entails habits of creating a coherent representation of the problem at hand; considering the units involved; attending to the meaning of quantities, not just how to compute them; and knowing and flexibly using different properties of operations and objects.
Math.Practice.MP3
| Common core State Standards
- Math: Math
- Practice: Mathematical Practice Standards
-
MP3: Construct viable arguments and critique the reasoning of others.
Mathematically proficient students understand and use stated assumptions, definitions, and previously established results in constructing arguments. They make conjectures and build a logical progression of statements to explore the truth of their conjectures. They are able to analyze situations by breaking them into cases, and can recognize and use counterexamples. They justify their conclusions, communicate them to others, and respond to the arguments of others. They reason inductively about data, making plausible arguments that take into account the context from which the data arose. Mathematically proficient students are also able to compare the effectiveness of two plausible arguments, distinguish correct logic or reasoning from that which is flawed, and--if there is a flaw in an argument--explain what it is. Elementary students can construct arguments using concrete referents such as objects, drawings, diagrams, and actions. Such arguments can make sense and be correct, even though they are not generalized or made formal until later grades. Later, students learn to determine domains to which an argument applies. Students at all grades can listen or read the arguments of others, decide whether they make sense, and ask useful questions to clarify or improve the arguments.
Math.4.NF.B.4b
Common core State Standards
- Math: Math
- 4: Grade 4
- NF: Number & Operations--Fractions
- B: Build fractions from unit fractions
-
4b:
Apply and extend previous understandings of multiplication to multiply a fraction by a whole number.
a. Understand a fraction a/b as a multiple of 1/b. For example, use a visual fraction model to represent 5/4 as the product 5 × (1/4), recording the conclusion by the equation 5/4 = 5 × (1/4).
b. Understand a multiple of a/b as a multiple of 1/b, and use this understanding to multiply a fraction by a whole number. For example, use a visual fraction model to express 3 × (2/5) as 6 × (1/5), recognizing this product as 6/5. (In general, n × (a/b) = (n × a)/b.)
c. Solve word problems involving multiplication of a fraction by a whole number, e.g., by using visual fraction models and equations to represent the problem. For example, if each person at a party will eat 3/8 of a pound of roast beef, and there will be 5 people at the party, how many pounds of roast beef will be needed? Between what two whole numbers does your answer lie?
<br />
Grade 4 expectations in this domain are limited to fractions with denominators 2, 3, 4, 5, 6, 8, 10, 12, 100.
Save to My Resources
PLEASE CREATE A NEW ACCOUNT OR LOG IN TO ACCESS THIS CONTENT
Enjoy your first video for free. Subscribe for unlimited access.
Have questions about subscribing?
Click Here to learn more about individual subscriptions.
Click Here to learn more about School and Institution access.
Discussion and Supporting Materials
Thought starters
- How did Ms. Spies create opportunities for students to share and learn from others?
- What did students learn by critiquing the teacher's solutions?
- How did Ms. Spies help her students further their understanding of multiplication as repeated addition?
School Details
Cypress Creek Elementary School6100 South Williamson Boulevard
Port Orange FL 32128
Population: 802
Data Provided By:

Teachers
Amy Spies
Newest
|
4 MIN
|
5 MIN
|
5 MIN
UNCUT CLASSROOMS
| TCHERS' VOICE
English Language Arts
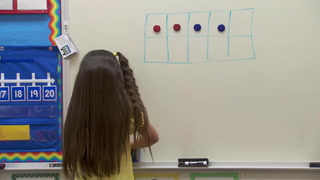












36 Comments
Troy Parsons Apr 27, 2019 6:31pm
I really enjoyed this video. It was nice to finally see one where things didn't go quite as planned (something that happens daily in teachers' classrooms). That was where I picked up some new ideas from you. I liked that when the kids weren't "getting" what you wanted them to know, you gave them "teacher models" on how to solve the problem and had the students critique them. It's always hard for students to find errors in their own work, but much easier to find it in someone else's work. Making this a "real world" example was also very good instead; it made me think about using more real examples when introducing new concepts, instead of straight to numbers.
Vanessa Costello Nov 24, 2016 10:59pm
Brooke Tunget Aug 4, 2016 4:55pm
Mary Bernhardt Mar 3, 2015 4:47pm
Valerie Robertson Feb 21, 2015 5:09pm