Series Engaging Students with "Productive Struggle": Deepening Understanding: Linear Equations
Math.A.CED.2
| Common core State Standards
- Math: Math
- A: Algebra
- CED: Creating Equations
-
2:
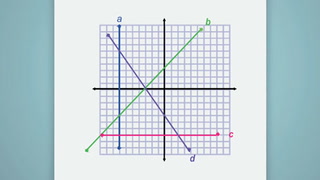
Create equations in two or more variables to represent relationships
between quantities; graph equations on coordinate axes with labels
and scales.
Math.A.REI.1
| Common core State Standards
- Math: Math
- A: Algebra
- REI: Reasoning with Equations and Inequalities
-
1:
Explain each step in solving a simple equation as following from the
equality of numbers asserted at the previous step, starting from the
assumption that the original equation has a solution. Construct a
viable argument to justify a solution method.
Math.Practice.MP2
Common core State Standards
- Math: Math
- Practice: Mathematical Practice Standards
-
MP2: Reason abstractly and quantitatively.
Mathematically proficient students make sense of quantities and their relationships in problem situations. They bring two complementary abilities to bear on problems involving quantitative relationships: the ability to decontextualize--to abstract a given situation and represent it symbolically and manipulate the representing symbols as if they have a life of their own, without necessarily attending to their referents—and the ability to contextualize, to pause as needed during the manipulation process in order to probe into the referents for the symbols involved. Quantitative reasoning entails habits of creating a coherent representation of the problem at hand; considering the units involved; attending to the meaning of quantities, not just how to compute them; and knowing and flexibly using different properties of operations and objects.
Save to My Resources
PLEASE CREATE A NEW ACCOUNT OR LOG IN TO ACCESS THIS CONTENT
Enjoy your first video for free. Subscribe for unlimited access.
Have questions about subscribing?
Click Here to learn more about individual subscriptions.
Click Here to learn more about School and Institution access.
Discussion and Supporting Materials
Thought starters
- What do the teachers discover during their review of the post assessment?
- How does formative assessment inform future instruction?
- Why does Ms. Morehead ask her students to make predictions?
Teachers
Susie Morehead
Jenny Barrett
Newest
|
4 MIN
|
5 MIN
|
5 MIN
UNCUT CLASSROOMS
| TCHERS' VOICE
English Language Arts


















8 Comments
Megan Wooldridge Mar 29, 2016 9:23pm
roger connor Feb 9, 2016 12:03pm
Gretchen Vierstra Jul 21, 2015 5:12pm
Blake Boffa Jul 21, 2015 4:31pm
Gretchen Vierstra Mar 5, 2015 3:24pm